The aim of this blogpost is to prove that given $n$ points with distinct $x$ coordinates there is a unique polynomial function passing through all of them. Basically this polynomial is the (unique) polynomial of lowest degree that ‘fits’ given data. This theroem is known as the ‘Lagrange interpolation theorem’ in numerical analysis.
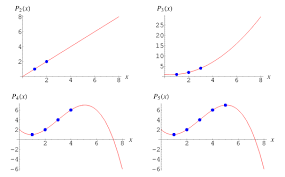
From hence forth by a set of $n$-coordinate pairs we refer to a set indexed by $0,1,\cdots,n-1$, such that the $x$-coordinates of all tuples in the set are distinct. It is well know that given a set of $2$-coordinate pairs one can find a unique linear function(a degree one polynomial) passing through them. Lagrange interpolation is an obvious generalization to this.
We first prove the existence part of this theorem. For a particular $k\leq n-1$ consider the set of $n$-coordinate pairs(we call sets with the aftermentioned property ‘special’), such that $(x_{k},1)$ belongs to the set and for all $i\leq n-1$ and $i\neq k$, $(x_{i},0)$ belongs to the set. Now we can construct the polynomial function: $$
f_{k}(x) = \frac{(x-x_0)}{(x_k-x_0)} \cdots \frac{(x-x_{k-1})}{(x_k-x_{k – 1})} \frac{(x-x_{k+1})}{(x_k-x_{k+1})} \cdots \frac{(x-x_{n-1})}{(x_j-x_{n-1})} =\prod_{0\le m\le n-1\ m\neq k} \frac{x-x_m}{x_k-x_m}$$
Clearly this function of degree $n-1$, fits the special $n$-coordinate sets for all $k$. Now given any $n$-coordinate set,$X$, for all $x$-coordinates, $x_{k}$, consider the function $f_{k}$ corresponding to the special set of $n$-coordinate pairs, such that $(x_{k},1)$ belongs to the pair. Through these $f_{k}$’s we can construct a polynomial function: $y_0f_0+y_1f_1\cdots+y_{n-1}f_{n-1}$ where $y_{m}’s$ are the $y$-coordinates of $X$ it’s easy to see that this is a $n-1$ degree polynomial function which fits $X$.
Uniqueness can be proven by algebra.
bx1v8b
ΠΠ»Π΅Π³Π°Π½ΡΠ½ΠΎΡΡΡ ΠΈ ΡΡΠΈΠ»Ρ Π² ΠΊΠ°ΠΆΠ΄ΠΎΠΉ Π΄Π΅ΡΠ°Π»ΠΈ.
ΠΠ΅Π±Π΅Π»Ρ ΠΏΡΠ΅ΠΌΠΈΡΠΌ https://byfurniture.by .
ΠΠ΄Π΅ΠΈ Π΄Π»Ρ ΠΎΡΠΎΡΠΌΠ»Π΅Π½ΠΈΡ Π΄ΠΈΠ·Π°ΠΉΠ½Π΅ΡΡΠΊΠΎΠΉ ΠΌΠ΅Π±Π΅Π»ΡΡ.
ΠΠΈΠ·Π°ΠΉΠ½Π΅ΡΡΠΊΠ°Ρ ΠΌΠ΅Π±Π΅Π»Ρ ΠΏΡΠ΅ΠΌΠΈΡΠΌ-ΠΊΠ»Π°ΡΡΠ° https://byfurniture.by .
https://honda-fit.ru/forums/index.php?autocom=gallery&req=si&img=7034
Π’ΠΎΠΏ Π½Π°ΠΏΡΠ°Π²Π»Π΅Π½ΠΈΡ Π΄Π»Ρ ΠΎΡΠ΄ΡΡ Π° Π² ΡΡΠΎΠΏΠΈΠΊΠ°Ρ .
ΠΠΊΠ·ΠΎΡΠΈΡΠ½ΠΈ ΠΏΠΎΡΠΈΠ²ΠΊΠΈ ΠΠΊΠ·ΠΎΡΠΈΡΠ½ΠΈ ΠΏΠΎΡΠΈΠ²ΠΊΠΈ .
ΠΠ±Π·ΠΎΡΡ ΠΏΠΎΠΏΡΠ»ΡΡΠ½ΡΡ Π°Π½ΡΠΈΡΡΡΠ΅ΡΡ ΠΈΠ³ΡΡΡΠ΅ΠΊ.
ΠΠ½ΡΠΈΡΡΡΠ΅Ρ ΡΠΈΠ΄ΠΆΠ΅Ρ ΠΈΠ³ΡΠ°ΡΠΊΠΈ https://www.antistres-igrachki.com/ .
ΠΡΠΊΠ»Ρ Π±Π΅Π±Π΅ΡΠ° β Π»ΡΡΡΠΈΠ΅ Π΄ΡΡΠ·ΡΡ Π²Π°ΡΠ΅Π³ΠΎ ΡΠ΅Π±Π΅Π½ΠΊΠ°.
ΠΠ΅ΠΊΠΈ ΠΊΡΠΊΠ»ΠΈ Π±Π΅Π±Π΅ΡΠ° http://www.kukli-bebeta.com/ .
ΠΠ½Π½ΠΎΠ²Π°ΡΠΈΠΎΠ½Π½ΡΠ΅ ΡΠ΅ΡΠ΅Π½ΠΈΡ Π΄Π»Ρ ΠΈΠΌΠΏΠ»Π°Π½ΡΠ°ΡΠΈΠΈ Π½Π° 4 ΠΈΠΌΠΏΠ»Π°Π½ΡΠ°Ρ .
ΠΠΌΠΏΠ»Π°Π½ΡΠ°ΡΠΈΡ 4 all ΠΏΠΎΠ΄ ΠΊΠ»ΡΡ http://www.belfamilydent.ru/services/implantaciya-allon4 .
ΠΡΠ΅ΠΈΠΌΡΡΠ΅ΡΡΠ²Π° Π·ΡΠ±Π½ΡΡ ΠΌΠΎΡΡΠΎΠ² ΠΏΠ΅ΡΠ΅Π΄ ΠΈΠΌΠΏΠ»Π°Π½ΡΠ°ΠΌΠΈ.
Π‘ΠΊΠΎΠ»ΡΠΊΠΎ ΡΡΠΎΠΈΡ ΠΌΠΎΡΡΠΎΠ²ΠΈΠ΄Π½ΡΠΉ ΠΏΡΠΎΡΠ΅Π· koronki-blog.ru/mostovidnoe-protezirovanie .
ΠΡΠΎΠ±Π΅Π½Π½ΠΎΡΡΠΈ ΡΡ ΠΎΠ΄Π° Π·Π° ΠΌΠΎΡΡΠΎΠ²ΠΈΠ΄Π½ΡΠΌΠΈ Π½Π΅ΡΡΠ΅ΠΌΠ½ΡΠΌΠΈ ΠΏΡΠΎΡΠ΅Π·Π°ΠΌΠΈ.
ΠΠΎΡΡΠΎΠ²ΠΈΠ΄Π½ΡΠΉ ΠΏΡΠΎΡΠ΅Π· Π½Π° Π²Π΅ΡΡ Π½ΡΡ ΡΠ΅Π»ΡΡΡΡ http://belfamilydent.ru/services/mostovidnoe-protezirovanie/ .
Π‘ΠΎΠ²ΡΠ΅ΠΌΠ΅Π½Π½ΡΠ΅ ΠΌΠ΅ΡΠΎΠ΄Ρ SEO.
Π Π°ΡΠΊΡΡΡΠΊΠ° ΡΠ°ΠΉΡΠΎΠ² ΡΠ°ΡΡΠ½ΡΠΉ ΠΌΠ°ΡΡΠ΅Ρ Π Π°ΡΠΊΡΡΡΠΊΠ° ΡΠ°ΠΉΡΠΎΠ² ΡΠ°ΡΡΠ½ΡΠΉ ΠΌΠ°ΡΡΠ΅Ρ .
ΠΠ°ΠΊ ΡΠ΄Π΅Π»Π°ΡΡ ΠΈΠ½ΡΠ΅ΡΡΠ΅Ρ ΡΡΠΎΠ½ΡΠ΅Π½Π½ΡΠΌ Ρ ΠΏΠΎΠΌΠΎΡΡΡ Π΄ΠΈΠ·Π°ΠΉΠ½Π΅ΡΡΠΊΠΈΡ ΡΠ»Π΅ΠΌΠ΅Π½ΡΠΎΠ².
ΠΠΈΠ·Π°ΠΉΠ½Π΅ΡΡΠΊΠ°Ρ ΠΌΠ΅Π±Π΅Π»Ρ ΠΏΡΠ΅ΠΌΠΈΡΠΌ-ΠΊΠ»Π°ΡΡΠ° http://www.byfurniture.by .